a 3

Use the Square Root Property on the binomial. The quadratic formula covering all cases was first obtained by Simon Stevin in 1594. 26, 2

x The roots of the quadratic equation are calculated by taking the square root of RHS. u 16

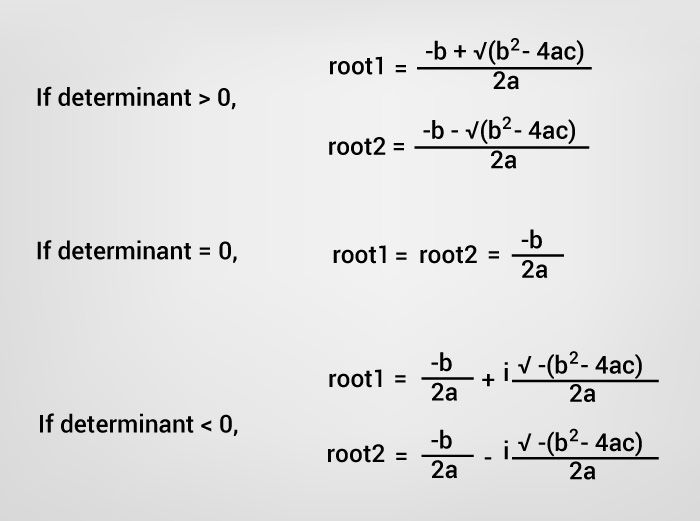
x 81, 64 2 r In a quadratic equation \ (a {x^2} + bx + c = 0,\) we get two equal real roots if \ (D = {b^2} 4ac = 0.\) In the graphical representation, we can see that the graph of The more general case where a does not equal 1 can require a considerable effort in trial and error guess-and-check, assuming that it can be factored at all by inspection. 9

+ x ) Parlez-en ! b z This must be addressed quickly because topics you do not master become potholes in your road to success. 2 It is sometimes convenient to reduce a quadratic equation so that its leading coefficient is one. Simplify: 128.128. 6 2 If the discriminant is positive there are two solutions, if negative there is no solution, if equlas 0 there is 1 solution. 2 In this case, a binomial is being squared. 1 2 The left sides of the equations in the next two examples do not seem to be of the form a(x h)2. It is written in the form: ax^2 + bx + c = 0 where x is the variable, and a, b, and c are constants, a 0.



25 Let's denote those roots \displaystyle\alpha and \displaystyle\beta , as follows: 2 2 =

+ =

1 4 = with some help. in the formula should be understood as "either of the two elements whose square is b2 4ac, if such elements exist". 30 45 {\displaystyle \sin 2\theta _{p}=-2{\frac {\sqrt {ac}}{b}},}, where the subscripts n and p correspond, respectively, to the use of a negative or positive sign in equation [1]. ) = Pair of Linear Equations in Two Variables . +

[36], If the quadratic equation 1
68 46 2 64 Methods of numerical approximation existed, called prosthaphaeresis, that offered shortcuts around time-consuming operations such as multiplication and taking powers and roots.

) = 2 y

the values of x x where this equation is solved. {\displaystyle b>0}

Solve Using the Quadratic Formula 5x2-7x-3=0 In the days before calculators, people would use mathematical tableslists of numbers showing the results of calculation with varying argumentsto simplify and speed up computation.

We have already solved some quadratic equations by factoring.

24

( 8 = x WebAnswer: Far be it for me to determine what people mean when they use, misuse, and abuse language concerning mathematics! 8



0 2 2

0 48 72

) t 0 = p 16 2 In the following exercises, solve each equation.

But what happens when we have an equation like x2 = 7?
Solve a quadratic equation using the square root property.

(

n In this case, the subtraction of two nearly equal numbers will cause loss of significance or catastrophic cancellation in the smaller root. + A quadratic equation is an equation of the form ax2 + bx + c = 0, where a0a0. )

4 8 u = 0. 1 2

+ 2

Only if it can be put in the form ax2 + bx + c = 0, and a is not zero.

We reviewed their content and use your feedback to 24 b q +

There can be 0, 1 or 2 solutions to a quadratic equation. + 2



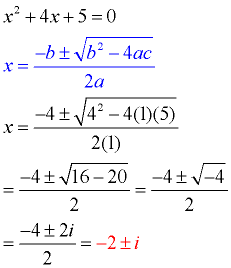
WebThe discriminant of the quadratic equation x 2 ( 5 k) x + ( k + 2) = 0 is = k 2 14 k + 17.





Now, Given and are roots of a quadratic equation x = and x = Conclusion: (x - )( x - )=0 ( + ) + = S.O.R = b a P.O.R = c a 5. {\displaystyle \theta =\cos ^{-1}\left({\tfrac {-b}{2{\sqrt {ac}}}}\right). x 2

Thus the x-coordinate of the vertex is, The y-coordinate can be obtained by substituting the above result into the given quadratic equation, giving, These formulas for the vertex can also deduced directly from the formula (see Completing the square). = 2 n But, the discriminant of is = ( 14) 2 4.17 = 128 > 0 is positive. When solving quadratic equations, the term \ ( {b^2} 4ac\) is used. 2 1 View solution.

If this cuts the middle line AB of the three then the equation has a solution, and the solutions are given by negative of the distance along this line from A divided by the first coefficient a or SA. The solutions to some equations may have fractions inside the radicals. 2 3 Form a quadratic b +

55 Question 4. +

75 x x 3 Transcript. 77 Factored Form: y=a (x-r_1) (x-r_2) y = a(x r1)(xr2) 3.

0, v When a quadratic equation's discriminant is zero, it has only one real root. 4 In the case that b 0, there are two distinct roots, but if the polynomial is irreducible, they cannot be expressed in terms of square roots of numbers in the coefficient field. If the parabola does not intersect the x-axis, there are two complex conjugate roots.

After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section. 2

1 + and 25 {\displaystyle x={\sqrt {c/a}}\tan \theta }, and then multiplying through by cos2() / c, we obtain, [3] 2 + Choose how would you respond to the statement I can solve quadratic equations of the form a times the square of x minus h equals k using the Square Root Property. Confidently, with some help, or No, I dont get it.. Euclid, the Greek mathematician, produced a more abstract geometrical method around 300 BC.

+ 32, 25

x 24 =

Standard Form: y=ax^2+bx+c y = ax2 +bx+ c 2. c

p

=

[26] He also described the method of completing the square and recognized that the discriminant must be positive,[26][27]:230 which was proven by his contemporary 'Abd al-Hamd ibn Turk (Central Asia, 9th century) who gave geometric figures to prove that if the discriminant is negative, a quadratic equation has no solution. 4, ( This is a special case of ArtinSchreier theory. Vieta's formulas (named after Franois Vite) are the relations, between the roots of a quadratic polynomial and its coefficients. 9.3: Solve Quadratic Equations by Completing the Square, Solve Quadratic Equations of the form ax2=kax2=k using the Square Root Property, How to solve a Quadratic Equation of the form ax2 = k Using the Square Root Property, Solving Quadratic Equations: The Square Root Property, Using the Square Root Property to Solve Quadratic Equations, source@https://openstax.org/details/books/intermediate-algebra-2e, status page at https://status.libretexts.org.

0, y + Inspirational Lessons Learned From Martin Luther King Jr. = 7 Factor: 9x212x+49x212x+4. The first step, like before, is to isolate the term that has the variable squared. 7 10 16 ) + 7 There is only one solution (and one root). A quadratic equation has two solutions if the discriminant b^2 - 4ac is positive. 2 2

1 ) ( v 2 9 2 WebThe quadratic function is a second order polynomial function: f ( x) = ax2 + bx + c The solutions to the quadratic equation are the roots of the quadratic function, that are the intersection points of the quadratic function graph with the x-axis, when f ( x) = 0 3 When the Discriminant ( b24ac) 11 14 So, every positive number has two square rootsone positive and one negative. requiring a and c to have the same sign as each otherthen the solutions for the roots can be expressed in polar form as[37], where These solutions may be both real, or both complex. When this happens, we must rationalize the denominator. in equation a x 2 + b x + c the roots will be equal if D = b 2 4 a Learn faster and smarter from top experts, Download to take your learnings offline and on the go.

= -19. For equations with real solutions, you can use the graphing tool to visualize the solutions. )

3 Protters & Morrey: "Calculus and Analytic Geometry. 64 and then inverting. WebThe value of k for which the quadratic equation k x 2 + 1 = k x + 3 x 11 x 2 has real and equal roots are Q. ) On the other hand, the polynomial x2 + ax + 1 is irreducible over F4, but it splits over F16, where it has the two roots ab and ab + a, where b is a root of x2 + x + a in F16.

4 ( x 36 = 2 20

2 ) For equations with real solutions, you can use the graphing tool to visualize the solutions. {\displaystyle r={\sqrt {\tfrac {c}{a}}}} 2 v

Notice that the quadratic term, x, in the original form ax2 = k is replaced with (x h). Language links are at the top of the page across from the title. 0, 4 + x

[33] In 1637 Ren Descartes published La Gomtrie containing the quadratic formula in the form we know today. = a , The quadratic function may be rewritten, Let d be the distance between the point of y-coordinate 2k on the axis of the parabola, and a point on the parabola with the same y-coordinate (see the figure; there are two such points, which give the same distance, because of the symmetry of the parabola). In a quadratic equation \ (a {x^2} + bx + c = 0,\) there will be two roots, either they can be equal or unequal, real or unreal or imaginary. We will factor it first. 2 x

If |x2| << |x1|, then x1 + x2 x1, and we have the estimate: The second Vieta's formula then provides: These formulas are much easier to evaluate than the quadratic formula under the condition of one large and one small root, because the quadratic formula evaluates the small root as the difference of two very nearly equal numbers (the case of large b), which causes round-off error in a numerical evaluation. {\displaystyle (c/a)/R} =

3 2

=

36

Cream City Brick For Sale, Kevin Paredes Age, Articles T